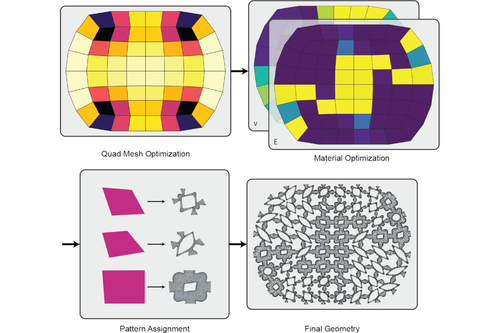
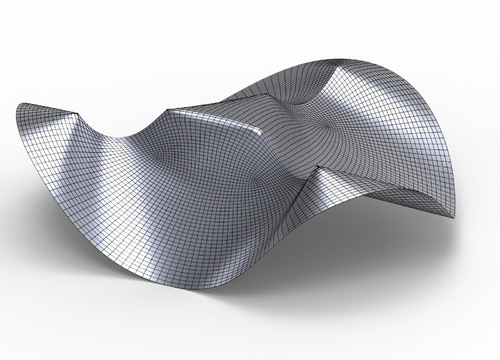
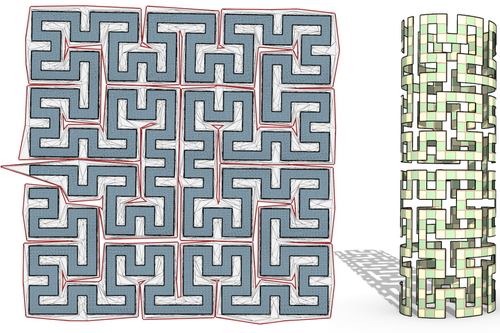
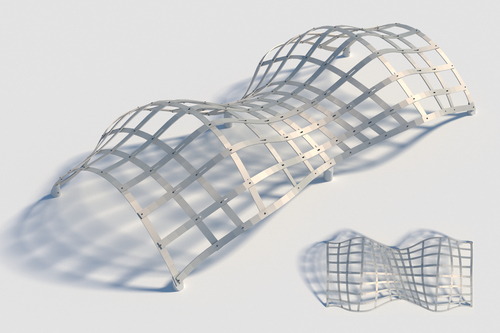
Abstract:
The isolines of principal symmetric surface parametrizations run symmetrically to the principal directions. We describe two discrete versions of these special nets/quad meshes which are dual to each other and show their usefulness for various applications in the context of fabrication and architectural design. Our discretization of a principal symmetric mesh comes naturally with a family of spheres, the so-called Meusnier and Mannheim spheres. In our representation of principal symmetric meshes, we have direct control over the radii of theses spheres and the intersection angles of the parameter lines. This facilitates tasks such as generating Weingarten surfaces including constant mean curvature surfaces and minimal surfaces. We illustrate the potential of Weingarten surfaces for paneling doubly curved freeform facades by significantly reducing the number of necessary molds. Moreover, we have direct access to curvature adaptive tool paths for cylindrical CNC milling with circular edges as well as flank milling with rotational cones. Furthermore, the construction of curved support structures from congruent circular strips is easily managed by constant sphere radii. The underlying families of spheres are in a natural way discrete curvature spheres in analogy to smooth Möbius and Laguerre geometry which further leads to a novel discrete curvature theory for principal symmetric meshes.